Mathematics is often perceived as a complex web of numbers and symbols, but at its core, it is a language that helps us make sense of the world. In Class 10, students are introduced to the intriguing concept of Pair of Linear Equations in Two Variables. This topic serves as a crucial foundation for understanding real-world scenarios and solving problems through mathematical reasoning. Let's embark on a journey to demystify this mathematical adventure.
Class 10 Mathematics (CBSE NCERT) – Chapter 3 Pair of Linear Equations in Two Variables
Pair of Linear Equations
Two Linear Equations having two same variables are known as the pair of Linear Equations in two variables.
-
a1x + b1y + c1 = 0
-
a2x + b2y + c2 = 0
Linear Equations in two variables are defined for a line which we can plot on a graph. The solutions to the linear equations are points. A linear equation in two variables, discussed in this chapter will form a straight line

Graphical Method of Solution of a Pair of Linear Equations
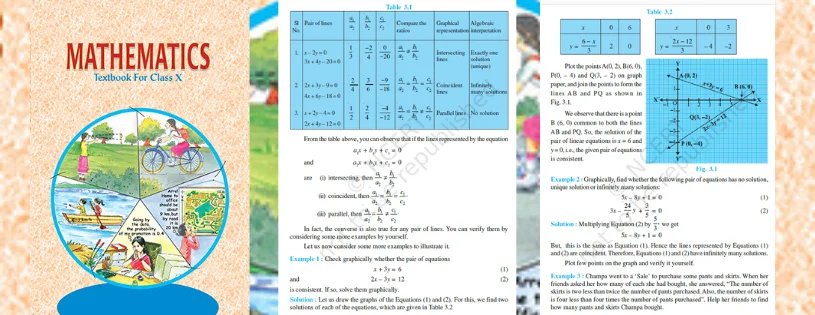
When two lines intersect each other at only one point, then we conclude that there is one and only one solution. It means that a unique solution exists for this pair of linear equations in two variables. This type of pair of linear equations is called a consistent pair of Linear equations.
If the two lines are coincident, we can say that the pair of linear equations will have infinitely many solutions. This type of pair of linear equations can be called an inconsistent pair of Linear equations.
If the two lines are parallel to each other, which means they do not meet at all, then we can say that the two linear equations will not have any common solution. This type of pair of linear equations will be called the dependent pair of Linear equations.
CBSE Class 10 NCERT Mathematics Topics for a Strong Foundation (NCERT DOWNLOAD)
| Chapter Name | Pair of Linear Equations in Two Variables |
| Topic Number | Topics |
| 3.1 | Introduction |
| 3.2 | Graphical Method of Solution of a Pair of Linear Equations |
| 3.3 | Algebraic Methods of Solving a Pair of Linear Equations |
| 3.4 | Substitution Method |
| 3.5 | Elimination Method |
| 3.6 | Summary |
Here, students are introduced to several important concepts that will be useful for those who wish to pursue mathematics
Geometric Interpretation
One fascinating aspect of pairs of linear equations is their geometric interpretation. These equations represent lines on a coordinate plane. The point where these lines intersect is the solution to the system. If the lines are parallel, there is no solution, and if they overlap, there are infinitely many solutions.
CBSE Class 10 Board Exam Sample Paper

[Previous Year Question Solution Maths Download Button]
[Previous Year Question Solution Science Download Button]
| Class 10 CBSE Mathematics Chapters |
| Chapter1: Real Numbers |
| Chapter2: Polynomials |
| Chapter3: Pair of Linear Equations in Two Variables |
| Chapter4: Quadratic Equations |
| Chapter5: Arithmetic Progressions |
| Chapter6: Triangles |
| Chapter7: Coordinate Geometry |
| Chapter8: Introduction to Trigonometry |
| Chapter9: Some Applications of Trigonometry |
| Chapter10: Circles |
| Chapter11: Areas Related to Circles |
| Chapter12: Surface Areas and Volumes |
| Chapter13: Statistics |
| Chapter14: Probability |
| Class 10 CBSE Science Chapters |
| Chapter1: Chemical Reactions and Equations |
| Chapter2: Acids, Bases and Salts |
| Chapter3: Metals and Non-metals |
| Chapter4: Carbon and its Compounds |
| Chapter5: Life Processes |
| Chapter6: Control and Coordination |
| Chapter7: How do Organisms Reproduce? |
| Chapter8: Heredity |
| Chapter9: Light – Reflection and Refraction |
| Chapter10: The Human Eye and the Colourful World |
| Chapter11: Electricity |
| Chapter12: Magnetic Effects of Electric Current |
| Chapter13: Our Environment |
| Class 8 |
| Class 9 |
| Class 11 |
| Class 12 |
CBSE Class 10th Downloadable Resources:
| 1. CBSE Class 10th Topic Wise Summary | View Page / Download |
| 2. CBSE Class 10th NCERT Books | View Page / Download |
| 3. CBSE Class 10th NCERT Solutions | View Page / Download |
| 4. CBSE Class 10th Exemplar | View Page / Download |
| 5. CBSE Class 10th Previous Year Papers | View Page / Download |
| 6. CBSE Class 10th Sample Papers | View Page / Download |
| 7. CBSE Class 10th Question Bank | View Page / Download |
| 8. CBSE Class 10th Topic Wise Revision Notes | View Page / Download |
| 9. CBSE Class 10th Last Minutes Preparation Resources (LMP) | View Page / Download |
| 10. CBSE Class 10th Best Reference Books | View Page / Download |
| 11. CBSE Class 10th Formula Booklet | View Page / Download |
Being in CBSE class 10th and considering the board examinations you must be needing resources to excel in your examinations. At TestprepKart we take great pride in providing CBSE class 10th all study resources in downloadable form for you to keep you going.
Below is the list of all CBSE class 10th Downloads available on TestprepKart for both Indian and NRI students preparing for CBSE class 10th in UAE, Oman, Qatar, Kuwait & Bahrain.
FAQ
Q1 Why are pair of linear equations important?
Ans They offer a powerful tool for modeling and solving real-world problems, from business scenarios to physics and engineering challenges.
Q2 How do you graphically interpret a pair of linear equations?
Ans The solution to the system of equations is represented by the point where the corresponding lines intersect on a coordinate plane.
Q3 What are the three primary methods for solving pairs of linear equations?
Ans Substitution Method, Elimination Method, and Cross-Multiplication Method.
Q4 Can a system of linear equations have no solution?
Ans Yes, if the lines represented by the equations are parallel and never intersect.
Q5 Is it possible for a system of linear equations to have infinite solutions?
Ans Yes, if the lines represented by the equations overlap, there are infinitely many solutions.
Q6 How does the Substitution Method work?
Ans It involves solving one equation for one variable and substituting that expression into the other equation.
Leave a Reply