Triangles, the fundamental shapes in geometry, showcase a mesmerizing dance of similarity. In this blog post, we embark on a captivating journey into the world of triangle similarity, unraveling its principles, applications, and the profound role it plays in shaping our understanding of proportions in geometry.
Geometry Unveiled The Symphony of Triangle Similarity and Proportional Precision
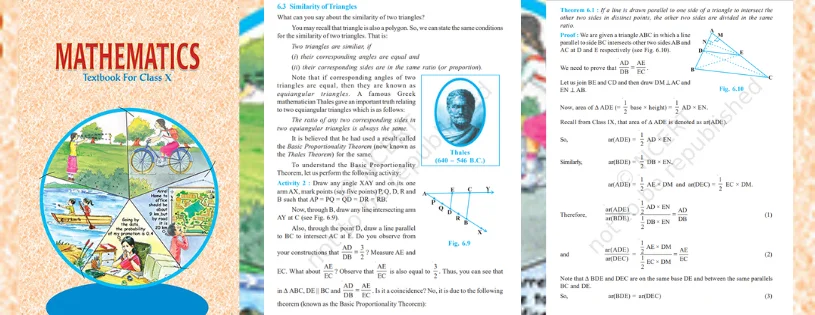
What is the Similarity of triangles?
The similarity of triangles can be defined based on their properties. Two triangles are similar, if
(i) their corresponding angles are equal and
(ii) their corresponding sides are in the same ratio (or proportion).
Criterion for Similarity of Triangles
The criterion of similarity of triangles include
-
AAA similarity criterion
-
AA similarity criterion
-
SSS similarity criterion
-
SAS similarity criterion
All these criteria are used to solve many triangle problems in maths. Let us understand the meaning of the similarity criterion of triangles here.
Understanding Triangle Similarity
Similar triangles share identical shapes but may differ in size. Unravel the principles governing their similarity through the examination of corresponding angles and proportional side lengths.
Key Theorems
Delve into the essential theorems that dictate triangle similarity, such as the Angle-Angle (AA) and Side-Angle-Side (SAS) theorems. Explore how these theorems form the basis for establishing triangles as akin geometric entities.
Real-World Applications
Triangle similarity extends beyond the confines of textbooks, finding applications in fields like architecture, photography, and navigation. Discover how architects use similar triangles to design structures, photographers apply it for perspective, and navigators employ it for distance calculations.
Ratios and Proportions
Unlock the power of ratios and proportions within similar triangles. Explore how the lengths of corresponding sides form consistent ratios, providing a versatile tool for solving a myriad of mathematical and real-world problems.
Problem-Solving Adventures
Engage in problem-solving adventures that challenge your understanding of triangle similarity. From determining unknown side lengths to solving real-world scenarios, these challenges enhance your mathematical prowess and critical thinking skills.
Properties of Similar Triangles
Similar triangles have the following properties
-
Similar triangles have the same shape but are not of the same size.
-
Each corresponding pair of angles of the two similar triangles is equal.
-
The ratio of any pair of corresponding sides of similar triangles is the same.
-
The ratio of the area of a pair of triangles is equal to the ratio of the square of the measurements of any pair of the corresponding sides.
Similarity Triangle Theorems
Two triangles are said to be similar if any of the similarity triangle theorems are proved. These theorems are basically like a criterion for a pair of triangles to pass in order to be considered as a pair of similar triangles. These theorems let the student quickly identify whether a pair of triangles is similar in terms of geometry.
-
AAA Similarity Criterion If two triangles are equal, then they are similar. By equiangular, it means that the measurements of the corresponding angles of the two triangles are equal. It is also called the Angle Angle Similarity Theorem.
-
SAS Similarity Criterion If in two triangles, two pairs of corresponding sides are proportional and the included angles are equal then the two triangles are similar. It is also called the Side Angle Side Similarity Theorem.
-
SSS Similarity Criterion If the corresponding sides of two triangles are proportional, then they are similar. It is also called the Side Side Side Similarity Theorem.
CBSE Class 10th Downloadable Resources:
| 1. CBSE Class 10th Topic Wise Summary | View Page / Download |
| 2. CBSE Class 10th NCERT Books | View Page / Download |
| 3. CBSE Class 10th NCERT Solutions | View Page / Download |
| 4. CBSE Class 10th Exemplar | View Page / Download |
| 5. CBSE Class 10th Previous Year Papers | View Page / Download |
| 6. CBSE Class 10th Sample Papers | View Page / Download |
| 7. CBSE Class 10th Question Bank | View Page / Download |
| 8. CBSE Class 10th Topic Wise Revision Notes | View Page / Download |
| 9. CBSE Class 10th Last Minutes Preparation Resources (LMP) | View Page / Download |
| 10. CBSE Class 10th Best Reference Books | View Page / Download |
| 11. CBSE Class 10th Formula Booklet | View Page / Download |
Being in CBSE class 10th and considering the board examinations you must be needing resources to excel in your examinations. At TestprepKart we take great pride in providing CBSE class 10th all study resources in downloadable form for you to keep you going.
Below is the list of all CBSE class 10th Downloads available on TestprepKart for both Indian and NRI students preparing for CBSE class 10th in UAE, Oman, Qatar, Kuwait & Bahrain.
SAMPLE PRACTICE QUESTION
Q1: What does it mean for two triangles to be similar?
Ans: Two triangles are similar if their corresponding angles are congruent, and the lengths of their corresponding sides are in proportion.
Q2: How can you visually determine the similarity of triangles?
Ans: If two triangles have the same shape but possibly different sizes, and their angles are identical, the triangles are similar.
Q3: What is the significance of the Angle-Angle (AA) criterion in establishing similarity?
Ans: The Angle-Angle criterion states that if two angles of one triangle are congruent to two angles of another triangle, the triangles are similar.
Q4: How does the Side-Angle-Side (SAS) criterion contribute to proving the similarity of triangles?
Ans: The SAS criterion states that if two sides of one triangle are proportional to two sides of another triangle, and the included angle is congruent, the triangles are similar.
Q5: Can the Side-Side-Side (SSS) criterion alone prove the similarity of triangles?
Ans: No, the SSS criterion alone does not guarantee similarity. Two triangles may have equal side lengths, but their angles may not necessarily be congruent.

| CBSE CLASS 10 Mathematics Chapter |
| Chapter:1 Real Numbers |
| Chapter:2 Polynomials |
| Chapter:3 Pair of Linear Equations in Two Variables |
| Chapter:4 Quadratic Equations |
| Chapter:5 Arithmetic Progressions |
| Chapter:6 Triangles |
| > Similar Figures |
| Chapter:7 Coordinate Geometry |
| Chapter:8 Introduction to Trigonometry |
| Chapter:9 Some Applications of Trigonometry |
| Chapter:10 Circles |
| Chapter:11 Areas Related to Circles |
| Chapter:12 Surface Areas and Volumes |
| Chapter:13 Statistics |
| Chapter:14 Probability |
| CBSE CLASS 10 Science Chapter |
| Chapter:1 Chemical Reactions and Equations |
| Chapter:2 Acids, Bases and Salts |
| Chapter:3 Metals and Non-metals |
| Chapter:4 Carbon and its Compounds |
| Chapter:5 Life Processes |
| Chapter:6 Control and Coordination |
| Chapter:7 How do Organisms Reproduce? |
| Chapter:8 Heredity |
| Chapter:9 Light – Reflection and Refraction |
| Chapter:10 The Human Eye and the Colourful World |
| Chapter:11 Electricity |
| Chapter:12 Magnetic Effects of Electric Current |
| Chapter:13 Our Environment |
| Class 8 |
| Class 9 |
| Class 11 |
| Class 12 |
Leave a Reply