Welcome to the captivating world of physics, where concepts like the "Centre of Mass" serve as the gravitational core around which the understanding of motion and equilibrium revolves. In this exploration, we will unravel the essence of the Centre of Mass, delving into its significance, applications, and the intriguing principles that govern it.
Unlocking the Essence of CBSE: Downloading Wisdom on Centre of Mass
What is the Center of Mass?
A position that is defined relative to an object or a system of objects is known as the Centre of Mass of that object. In a system, the average position of all parts that are weighted according to their Masses is defined as the Centre of Mass. The Centre of Mass for the simple rigid objects having uniform density is located at the Centroid. It is possible that sometimes the Centre of Mass of an object does not lie over it. In the case of a uniform disc, the Centre of Mass would lie on its Centre, while the Centre of Mass in the case of a ring lies on the Centre but there is not any material.
The Centre of Mass for complicated shapes can be defined as the unique position where the sum of the weighted position vectors of all the parts equals zero.
Importance of Centre of Mass
The Centre of Mass of a system is that one point where any uniform force is acted upon the object. It is important to find the Centre of Mass of objects as it makes it easy to solve the Mechanics’ problems in order to describe the Motion of complicated and oddly shaped objects. While doing calculations, we assume that all the Mass of an oddly-shaped object is concentrated in a tiny object that is located at the Centre of Mass, and this tiny object that we have assumed is known as the point Mass.
The formula for the Centre of Mass
The Vector Addition of the weighted position vectors that point towards the Centre of Mass of each object, gives the Centre of Mass of that system. The Centre of Mass is calculated separately for the components along each axis.

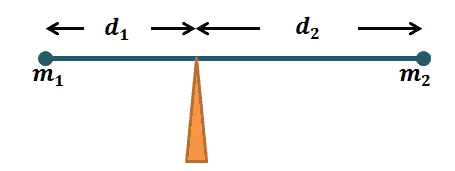
Center of Mass of a Two-Particle System
Let us consider a system of two particles of masses m1 and m2 located at points A and B respectively.
Let r1 and r2 be the position vectors of the particles relative to a fixed origin O. Then, the position vector rcm of the centre of mass C of the system is defined by (m1+m2) rcm =m1 r1+m2 r2.
The product of the total mass of the system and the position vector of the center of mass is equal to the sum of the products of the masses of the two particles and their respective position vectors.
Center of Gravity
The imaginary point through which on an object or a system, the force of Gravity is acted upon is known as the Centre of Gravity of that system. Usually, it is assumed while doing mechanical problems that the gravitational field is uniform which means that the Centre of Gravity and the Centre of Mass is at the same position.
Solved Example
Example 1: m₁=2 kg and m₂=5 kg are two-point Masses located at y₁=10m and y₂=-5m respectively. Calculate the Centre of Mass.

Example 2: In the HCL molecule, the separation between the nuclei of the two atoms is 1.27 A. Find the location of the centre of mass of the molecule. A chlorine atom is 35.5 times heavier than a hydrogen atom.
Solution: The centre of mass of the HCL molecule will be on the line joining H and CL atoms. Let the HCL molecule be along the X-axis, the H atom being at the origin (x=0). The centre of mass relative to the H atom is given by .

Example 3: Two bodies of masses 0.5 kg and 1 kg are lying in the X-Y plane at points (-1, 2) and (3,4) respectively. Locate the centre of mass of the system.
Solution : Here, we have

SAMPLE PACTICE QUESTIONS OF SIGNIFICANT FIGURES:
Q1. What is the Center of Mass (COM)?
Answer: The Center of Mass is a theoretical point representing the average position of mass in a system. It's where the entire mass can be treated as if it were concentrated, simplifying the analysis of the system's motion.
Q2. Why is the Center of Mass Important in Physics?
Answer: The concept of Center of Mass is crucial for simplifying the analysis of the motion of objects and systems. It allows us to treat the entire mass as if it were concentrated at a single point, making it easier to apply principles of motion.
Q3. Can the Center of Mass be Outside the Object?
Answer: Yes, the Center of Mass can be outside the physical boundaries of the object. It depends on the distribution of mass within the object. For irregularly shaped objects, the Center of Mass may not coincide with any part of the object itself.
Q4. How does the Center of Mass Influence Motion?
Answer: The Center of Mass behaves as if it were subjected to external forces. This allows us to analyse the motion of an object or a system more easily, applying Newton's laws of motion to the concentrated mass point.
Q5. Is the Center of Mass the Same as the Geometric Center?
Answer: Not necessarily. The Center of Mass is influenced by both the mass and its distribution, while the geometric centre is based solely on the shape. In asymmetrical distributions, the Center of Mass may not coincide with the geometric centre.

Leave a Reply