In the captivating world of geometry, the concept of similar figures opens a door to understanding the proportional relationships that govern shapes. This blog post embarks on a journey to explore the intricacies of similar figures, their properties, and the significance they hold in various mathematical and real-world contexts.
Proportional Harmony Navigating the World of Similar Figures in Geometry
What are Similar Figures?
Similar Figures are those figures which have the same shape, but the magnitude of their dimensions may or may not be equal. If the magnitude of their dimensions is also equal, then they are said to be congruent figures. All congruent figures are similar, but all similar figures are not congruent.
Similar Figures Definition
In terms of Maths, when two figures have the same shape but their sizes are different, then such figures are called similar figures. For example, different-sized photographs of a person i.e. stamp size, passport size, etc. depict similar objects but are not congruent. In geometry, two similar shapes such as similar triangles, similar rectangles, and similar squares, are the shapes whose dimensions are in equal or common ratio but the size or length of their sides vary. The common ratio is called the scale factor. Also, the corresponding angles are equal in measure.
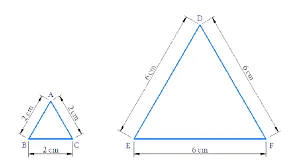
If two figures are similar then they are represented by the symbol ‘∼’. Let us say there are two triangles, ABC and PQR, which are similar, then they are represented as;
∆ABC ~ ∆ PQR
Now if the two triangles are similar to each then their corresponding sides should be in proportion. Hence,
- AB/PQ = BC/QR = AC/PR
- ∠A = ∠P, ∠B = ∠Q, ∠C = ∠R
Defining Similar Figures
Similar figures share the same shape but may have different sizes. Understanding the principles that govern their similarity involves exploring the ratios of corresponding sides and angles.
Properties and Ratios
Discover the key properties that make figures similar, including the equality of corresponding angles and proportional relationships between corresponding sides. The importance of these ratios in establishing similarity forms the foundation of this geometric exploration.
Similar Figures Area and Volume
If two figures are similar, then their corresponding sides are proportional. Or when can the ratio of their sides be equal?
Now, if we take the ratio of their surface areas, then it will be equal to the square of ratio of side. The ratio of the volume of two similar figures will be equal to the cube of the ratio of the length of sides.
Note: These are not the surface area and volume of the figures but the ratios.
Hence, based on the statements mentioned above, the scale factors of area and volume can be represented as;
SFA = SF2
SFV = SF3
where SFA is the scale factor of surface area and SFV is the scale factor of volume
Applications in Real Life
Similar figures find practical applications in fields such as architecture, art, and design. From creating scale models to determining proportions in artistic compositions, the concept of similarity bridges the gap between mathematical abstraction and real-world utility.
Scale Drawings and Maps
The blog delves into the world of scale drawings, where the principles of similar figures play a crucial role. Explore how architects and cartographers use these principles to represent objects and geographical features accurately.
Problem-solving and Critical Thinking
Engaging with similar figures involves solving problems that stimulate critical thinking. From determining unknown side lengths to solving real-world scenarios, these challenges enhance mathematical skills and problem-solving abilities.
CBSE Class 10th Downloadable Resources:
| 1. CBSE Class 10th Topic Wise Summary | View Page / Download |
| 2. CBSE Class 10th NCERT Books | View Page / Download |
| 3. CBSE Class 10th NCERT Solutions | View Page / Download |
| 4. CBSE Class 10th Exemplar | View Page / Download |
| 5. CBSE Class 10th Previous Year Papers | View Page / Download |
| 6. CBSE Class 10th Sample Papers | View Page / Download |
| 7. CBSE Class 10th Question Bank | View Page / Download |
| 8. CBSE Class 10th Topic Wise Revision Notes | View Page / Download |
| 9. CBSE Class 10th Last Minutes Preparation Resources (LMP) | View Page / Download |
| 10. CBSE Class 10th Best Reference Books | View Page / Download |
| 11. CBSE Class 10th Formula Booklet | View Page / Download |
Being in CBSE class 10th and considering the board examinations you must be needing resources to excel in your examinations. At TestprepKart we take great pride in providing CBSE class 10th all study resources in downloadable form for you to keep you going.
Below is the list of all CBSE class 10th Downloads available on TestprepKart for both Indian and NRI students preparing for CBSE class 10th in UAE, Oman, Qatar, Kuwait & Bahrain.
SAMPLE PRACTICE QUESTION
Q1: What are similar figures in geometry?
Ans: Similar figures are geometric shapes that have the same shape but may differ in size. Corresponding angles are equal, and corresponding sides are in proportion.
Q2: How can you determine if two figures are similar?
Ans: Two figures are similar if their corresponding angles are congruent, and the ratios of the lengths of their corresponding sides are equal.
Q3: What is the significance of corresponding angles in similar figures?
Ans: Corresponding angles in similar figures are equal, ensuring that the shapes have the same orientation and angular relationships.
Q4: In similar triangles, how do corresponding sides relate to each other?
Ans: Corresponding sides in similar triangles are in proportion. This means that the ratio of the length of one side to the length of the corresponding side in the other triangle is constant.
Q5: Can similar figures include different types of polygons, or are they limited to triangles?
Ans: Similar figures can include any polygons, not just triangles. As long as the angles are congruent, and the sides are in proportion, the figures are considered similar.

| CBSE CLASS 10 Mathematics Chapter |
| Chapter:1 Real Numbers |
| Chapter:2 Polynomials |
| Chapter:3 Pair of Linear Equations in Two Variables |
| Chapter:4 Quadratic Equations |
| Chapter:5 Arithmetic Progressions |
| Chapter:6 Triangles |
| > Similarity of Triangles |
| Chapter:7 Coordinate Geometry |
| Chapter:8 Introduction to Trigonometry |
| Chapter:9 Some Applications of Trigonometry |
| Chapter:10 Circles |
| Chapter:11 Areas Related to Circles |
| Chapter:12 Surface Areas and Volumes |
| Chapter:13 Statistics |
| Chapter:14 Probability |
| CBSE CLASS 10 Science Chapter |
| Chapter:1 Chemical Reactions and Equations |
| Chapter:2 Acids, Bases and Salts |
| Chapter:3 Metals and Non-metals |
| Chapter:4 Carbon and its Compounds |
| Chapter:5 Life Processes |
| Chapter:6 Control and Coordination |
| Chapter:7 How do Organisms Reproduce? |
| Chapter:8 Heredity |
| Chapter:9 Light – Reflection and Refraction |
| Chapter:10 The Human Eye and the Colourful World |
| Chapter:11 Electricity |
| Chapter:12 Magnetic Effects of Electric Current |
| Chapter:13 Our Environment |
| Class 8 |
| Class 9 |
| Class 11 |
| Class 12 |
Leave a Reply