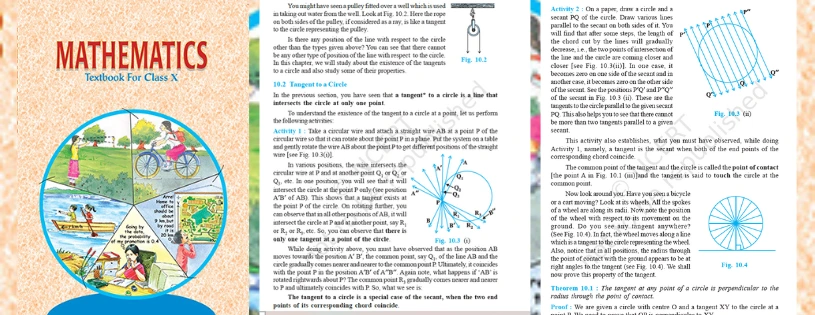
As we delve into the fascinating realm of geometry, the concept of tangents to a circle takes center stage. Understanding tangents not only enriches our grasp of circles but also opens the door to a myriad of applications in real-world scenarios. Join us on a journey through the curves and connections that define the relationship between circles and tangents.
Mastering Geometry: CBSE NCERT Download Unravels the Intricacies of Tangent to a Circle
Definition of Tangent to Circle
A line that joins two close points from a point on the circle is known as a tangent. In simple words, we can say that the lines that intersect the circle exactly in one single point are tangents. Only one tangent can be at a point to circle. The point where a tangent touches the circle is known as the point of tangency. The point where the circle and the line intersect is perpendicular to the radius. As it plays a vital role in the geometrical construction there are many theorems related to it which we will discuss further in this chapter.
(image will be uploaded soon)
Here, point O is the radius, point P is the point of tangency.
Various Conditions of Tangency
Only when a line touches the curve at a single point it is considered a tangent. Or else it is considered only to be a line. Hence, we can define tangent based on the point of tangency and its position with respect to the circle.
-
When point lies on the circle
-
When point lies inside the circle
-
When point lies outside the circle
Properties of Tangent
Always remember the below points about the properties of a tangent
-
A line of tangent never crosses the circle or enters it; it only touches the circle.
-
The point at which the lien and circle intersect is perpendicular to the radius
-
The tangent segment to a circle is equal from the same external point.
-
A tangent and a chord forms an angle, the angle is exactly similar to the tangent inscribed on the opposite side of the chord.
When Point Lies on the Circl
Here, from the figure, it is stated that there is only one tangent to a circle through a point that lies on the circle.
Equation of Tangent to a Circle
Below is the equation of tangent to a circle
-
Tangent to a circle equation x2+ y2=a2 at (a cos θ, a sin θ) is x cos θ+y sin θ= a
-
Tangent to a circle equation x2+ y2=a2 at (x1, y1) is xx1+yy1= a2
-
Tangent to a circle equation x2+ y2=a2 for a line y = mx +c is y = mx ± a √[1+ m2]
-
Tangent to a circle equation x2+ y2=a2 at (x1, y1) is xx1+yy1= a2
Tangent to a Circle Formula
To understand the formula of the tangent look at the diagram given below.
Here, we have a circle with P as its exterior point. From the exterior point P the circle has a tangent at Point Q and S. A straight line that cuts the curve in two or more parts is known as a secant. So, here the secant is PR and at point Q, R intersects the circle as shown in the diagram above. So, now we get the formula for tangent-secant
PR/PS = PS/ PQ
PS² = PQ.PR
I. The Basics: Unveiling the Tangent-Circle Connection
Before we explore the intricacies, let's establish the foundation. Tangents to a circle are lines that touch the circle at exactly one point, creating a right angle with the radius at the point of contact. This simple definition sets the stage for a deeper exploration of geometric relationships.
II. Tangents and Chords: A Dance of Symmetry
The connection between tangents and chords adds a layer of complexity to our geometric narrative. Students delve into the symmetrical relationships that emerge when tangents and chords intersect, unraveling the beauty of circle geometry.
III. Properties and Theorems: Decoding the Language of Tangents
As we progress, the blog explores essential properties and theorems related to tangents. From the Secant-Tangent Theorem to the Tangent-Tangent Angle Theorem, these insights empower students to navigate and solve geometric problems with confidence.
CBSE Class 10th Downloadable Resources:
| 1. CBSE Class 10th Topic Wise Summary | View Page / Download |
| 2. CBSE Class 10th NCERT Books | View Page / Download |
| 3. CBSE Class 10th NCERT Solutions | View Page / Download |
| 4. CBSE Class 10th Exemplar | View Page / Download |
| 5. CBSE Class 10th Previous Year Papers | View Page / Download |
| 6. CBSE Class 10th Sample Papers | View Page / Download |
| 7. CBSE Class 10th Question Bank | View Page / Download |
| 8. CBSE Class 10th Topic Wise Revision Notes | View Page / Download |
| 9. CBSE Class 10th Last Minutes Preparation Resources (LMP) | View Page / Download |
| 10. CBSE Class 10th Best Reference Books | View Page / Download |
| 11. CBSE Class 10th Formula Booklet | View Page / Download |
Being in CBSE class 10th and considering the board examinations you must be needing resources to excel in your examinations. At TestprepKart we take great pride in providing CBSE class 10th all study resources in downloadable form for you to keep you going.
Below is the list of all CBSE class 10th Downloads available on TestprepKart for both Indian and NRI students preparing for CBSE class 10th in UAE, Oman, Qatar, Kuwait & Bahrain.
SAMPLE PRACTICE QUESTION
Q1: What is a tangent to a circle?
Ans: A tangent to a circle is a straight line that touches the circle at a single point without intersecting it. This point of contact is known as the point of tangency.
Q2: How is the point of tangency determined for a tangent to a circle?
Ans: The point of tangency is where the tangent line and the circle meet. At this point, the radius drawn to the point is perpendicular to the tangent line.
Q3: What is the relationship between the radius and the tangent at the point of tangency?
Ans: The radius at the point of tangency is perpendicular to the tangent line. This relationship forms a right angle.
Q4: Can a circle have more than one tangent at a given point?
Ans: No, a circle has only one tangent at any given point on its circumference. The tangent is unique for each point of contact.
Q5: How does the length of the tangent relate to the radius of the circle?
Ans: The length of the tangent is equal to the radius of the circle. This relationship holds true for any tangent drawn to a circle.

Leave a Reply