The elastic behavior of materials is a fundamental aspect that influences their response to external forces. Understanding how materials deform and return to their original shape is crucial across various fields, from engineering to everyday applications. This phenomenon is a cornerstone in designing structures that withstand stress, creating durable products, and advancing technological innovations. Exploring the applications of elastic behavior unveils its significance in optimizing material performance, enhancing resilience, and contributing to the efficiency of diverse systems. In this blog, we will delve into the multifaceted applications that leverage the remarkable elasticity of materials for practical and innovative solutions.

Exploring the Diverse Applications of Elastic Behavior in Materials:
What Does Elasticity Mean?
Elasticity refers to the property of materials to deform under the influence of an external force and return to their original shape and size once the force is removed. In other words, a material can stretch or compress and then revert to its initial form. This characteristic is essential in understanding how materials respond to stress and strain. Elasticity is a fundamental concept in physics and engineering, playing a crucial role in designing structures, creating durable products, and advancing various technological applications. The study of elasticity provides insights into material behavior under different conditions, contributing to industry innovations.
What Do You Understand by the Elastic Behaviour of Materials?
The elastic behavior of materials refers to their ability to undergo deformation in response to an applied force and subsequently return to their original shape and size once the force is removed. When a material experiences stress (force applied) within its elastic limit, it deforms elastically. This means that the atoms or molecules in the material temporarily shift from their original positions but return to their initial arrangement when the force is released.
The elastic behavior is characterized by the material's ability to store and release energy during deformation, displaying a reversible response. This property is crucial in various fields such as engineering, physics, and materials science, as it influences the design and performance of structures, products, and devices. Understanding elastic behavior helps engineers and scientists optimize materials for durability, flexibility, and resilience in practical applications.
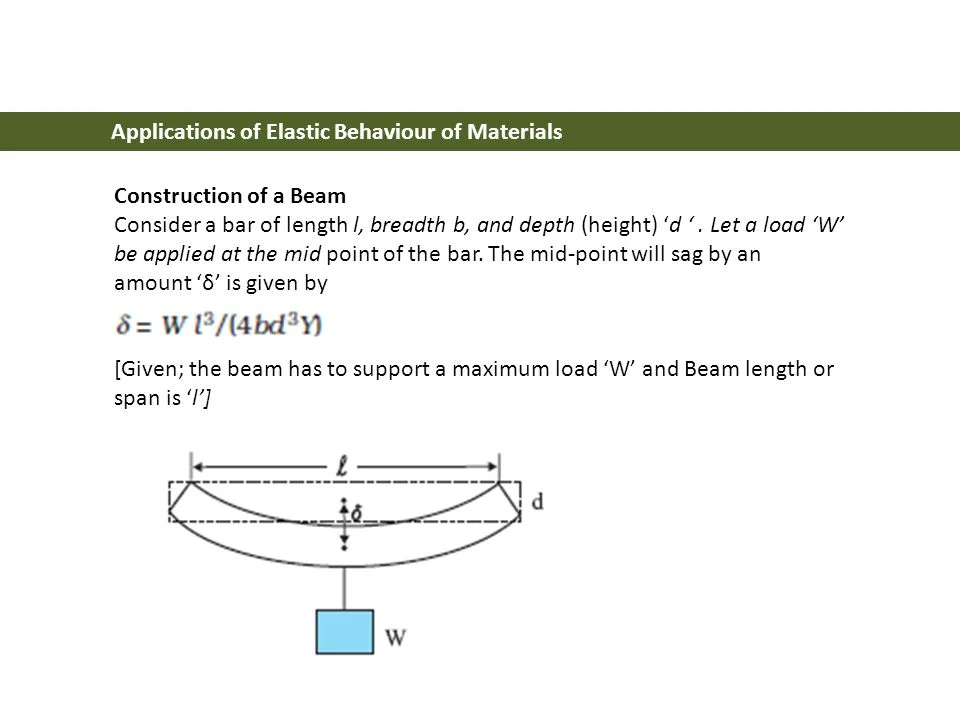
More on the Study of Elasticity
The study of elasticity delves into the intricate mechanics governing a material's response to external forces, providing invaluable insights for diverse applications. Key aspects of this field include:
-
Stress and Strain: Elasticity explores how materials react to stress (force applied) and strain (resulting deformation). Stress is the force applied per unit area, while strain is the change in shape or size relative to the original.
-
Hooke's Law: Named after physicist Robert Hooke, this foundational principle states that, within the elastic limit, stress is directly proportional to strain. This linear relationship is crucial for predicting material behavior under varying loads.
-
Elastic Modulus: Also known as Young's Modulus, Bulk Modulus, and Shear Modulus, these parameters quantify a material's stiffness under different types of deformation. They are essential for material characterization and selection in engineering design.
-
Elastic Limit: Every material has a threshold beyond which it undergoes permanent deformation. Understanding the elastic limit is critical for ensuring the integrity and longevity of structures and products.
-
Energy Storage and Release: Elastic deformation involves the storage of potential energy within a material, which is released when the material returns to its original state. This characteristic is exploited in various applications, from shock absorption to energy-efficient designs.
- Applications in Engineering: The principles of elasticity are fundamental in designing structures, machinery, and consumer products. Engineers use this knowledge to optimize the performance, safety, and durability of materials in real-world scenarios.
-
Material Testing: Experimental techniques, such as tensile testing and stress-strain analysis, are employed to assess a material's elastic properties. This information is crucial for quality control and ensuring materials meet specific design criteria.
-
Beyond Solids: Elasticity extends beyond traditional solid materials. Fluids, like gases and liquids, also exhibit elastic behavior, albeit with different characteristics. This understanding is crucial in fields like fluid dynamics and acoustics.
Example of Elasticity – Hooke’s Law
Hooke's Law, a fundamental principle in the study of elasticity, is best exemplified through the behavior of a spring. Consider a simple spring suspended from a fixed point. When a force (F) is applied to the lower end of the spring, it stretches. According to Hooke's Law, the force required to stretch or compress a spring is directly proportional to the displacement (change in length) of the spring from its equilibrium position.
Mathematically, Hooke's Law is expressed as:
F=−k⋅Δx
where:
-
F is the force applied,
-
k is the spring constant (a measure of the stiffness of the spring), and
-
Δx is the displacement from the equilibrium position.
The negative sign indicates that the force exerted by the spring is opposite to the direction of displacement.
For example, if a force of 10 Newtons is applied to a spring with a constant
k of 5 N/m (Newton per meter), and the spring stretches by 2 meters, you can use Hooke's Law to calculate the force:
F=−k⋅Δx
F=−5N/m⋅2m
F=−10N
So, in this case, a force of 10 Newtons is required to stretch the spring by 2 meters, by Hooke's Law. Importantly, this linear relationship holds as long as the material is within its elastic limit. Once the elastic limit is exceeded, the spring may undergo permanent deformation. Hooke's Law is widely applied in various fields, from engineering to physics, providing a foundational understanding of elastic behavior in materials.

CBSE Class 11th Downloadable Resources:
| 1. CBSE Class 11th Topic Wise Summary | View Page / Download |
| 2. CBSE Class 11th NCERT Books | View Page / Download |
| 3. CBSE Class 11th NCERT Solutions | View Page / Download |
| 4. CBSE Class 11th Exemplar | View Page / Download |
| 5. CBSE Class 11th Previous Year Papers | View Page / Download |
| 6. CBSE Class 11th Sample Papers | View Page / Download |
| 7. CBSE Class 11th Question Bank | View Page / Download |
| 8. CBSE Class 11th Topic Wise Revision Notes | View Page / Download |
| 9. CBSE Class 11th Last Minutes Preparation Resources | View Page / Download |
| 10. CBSE Class 11th Best Reference Books | View Page / Download |
| 11. CBSE Class 11th Formula Booklet | View Page / Download |
Being in CBSE class 11th and considering the board examinations, you must need resources to excel. At TestprepKart we take great pride in providing CBSE class 11th all study resources in downloadable form for you to keep you going.
Below is the list of all CBSE class 11th Downloads available on TestprepKart for both Indian and NRI students preparing for CBSE class 11th in UAE, Oman, Qatar, Kuwait & Bahrain.
SAMPLE PRACTICE QUESTIONS OF SIGNIFICANT FIGURES :
Q1. What is elasticity in materials?
Answer: Elasticity in materials refers to their ability to deform under an applied force and return to their original shape and size when the force is removed. It is a fundamental property that influences how materials respond to stress and strain.
Q2. How is elasticity measured?
Answer: Elasticity is often measured using parameters like Young's Modulus, Bulk Modulus, and Shear Modulus. Experimental methods, such as tensile testing, are employed to assess a material's response to stress and strain.
Q3. What is Hooke's Law, and how does it relate to elasticity?
Answer: Hooke's Law states that the force required to deform a material is directly proportional to the displacement. It is a fundamental principle in elasticity, especially for materials within their elastic limit.
Q4. What are the practical applications of elastic behavior in materials?
Answer: Elasticity is crucial in designing structures for civil engineering, creating resilient products in manufacturing, and developing medical devices like stents. It also plays a role in flexible electronics and various other innovative technologies.
Q5. How does the elastic limit affect material behavior?
Answer: The elastic limit is the maximum stress a material can endure while still returning to its original shape. Beyond this limit, permanent deformation may occur. Understanding the elastic limit is essential for designing materials to withstand specific conditions.

| Class 11th CBSE Physics Chapters |
| Chapter1: UNITS AND MEASUREMENTS |
| Chapter2: MOTION IN A STRAIGHT LINE |
| Chapter3: MOTION IN A PLANE |
| Chapter4: LAWS OF MOTION |
| Chapter5: WORK, ENERGY AND POWER |
| Chapter6: SYSTEM OF PARTICLES AND ROTATIONAL MOTION |
| Chapter7: GRAVITATION |
| Chapter8: MECHANICAL PROPERTIES OF SOLIDS |
| Chapter9: MECHANICAL PROPERTIES OF FLUIDS |
| Chapter10: THERMAL PROPERTIES OF MATTER |
| Chapter12: KINETIC THEORY |
| Chapter13: OSCILLATIONS |
| Chapter14: WAVES |
| Class 11th CBSE Chemistry Chapters |
| Chapter1: SOME BASIC CONCEPTS OF CHEMISTRY |
| Chapter2: STRUCTURE OF ATOMS |
| Chapter3: CLASSIFICATION OF ELEMENTS AND PERIODICITY IN PROPERTIES |
| Chapter4: CHEMICAL BONDING AND MOLECULAR STRUCTURE |
| Chapter5: THERMODYNAMICS |
| Chapter6: EQUILIBRIUM |
| Chapter7: REDOX REACTIONS |
| Chapter8: ORGANIC CHEMISTRY – SOME BASIC PRINCIPLE AND TECHNIQUES |
| Chapter9: Hydrocarbons HYDROCARBONS |
| Class 11th CBSE Mathematics chapter |
| Chapter1: SETS |
| Chapter2: RELATIONS AND FUNCTIONS |
| Chapter3: TRIGONOMETRIC FUNCTIONS |
| Chapter4: COMPLEX NUMBER AND QUADRATIC EQUATIONS |
| Chapter5: LINEAR INEQUALITIES |
| Chapter6: PERMUTATIONS AND COMBINATIONS |
| Chapter7: BINOMIAL THEOREM |
| Chapter8: SEQUENCES AND SERIES |
| Chapter9: STRAIGHT LINES |
| Chapter10: CONIC SECTIONS |
| Chapter11: INTRODUCTION TO THREE-DIMENSIONAL GEOMETRY |
| Chapter12: LIMITS AND DERIVATIVES |
| Chapter13: STATISTICS |
| Chapter14: PROBABILITY |
| Class 8 Link soon |
| Class 9 Link soon |
| Class 10 Link soon |
| Class 12 Link soon |
Leave a Reply