Vectors play a crucial role in various fields, from physics to computer science, offering a powerful way to represent and analyse quantities with both magnitude and direction. One fundamental operation with vectors is the multiplication by real numbers. In this blog post, we'll delve into the concept of multiplying vectors by real numbers, exploring its significance, properties, and real-world applications.
Understanding the Multiplication of Vectors by Real Numbers.
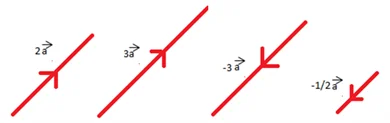
The Basics of Vector Multiplication
Defining Scalar Multiplication
Scalar multiplication is the process of multiplying a vector by a scalar, which is simply a real number. Let's denote a vector as v and a scalar as c. The result of scalar multiplication is a new vector, often denoted as c v. The key idea is that each component of the original vector is multiplied by the scalar:

c[v1v2⋮vn]=[c⋅v1c⋅v2⋮c⋅vn]
Significance in Scaling
Scalar multiplication scales the vector. If the scalar is greater than 1, the resulting vector is longer (in the same direction); if it's between 0 and 1, the vector is shorter. If the scalar is negative, the vector reverses direction.
Properties of Scalar Multiplication
Understanding the properties of scalar multiplication provides insights into its behaviour and application.
1. Distributive Property
Scalar multiplication is distributive over vector addition. For vectors u and v and a scalar c:
c(u+v)=c⋅u+c⋅v
This property allows for simplifying expressions involving scalar multiplication and vector addition.
2. Associative Property
Scalar multiplication is associative with respect to both scalar and vector multiplication:
(a⋅b)⋅v=a⋅(b⋅v)
This property enables flexibility in how scalar multiplication is applied.
3. Identity Element
Multiplying a vector by the scalar 1 leaves the vector unchanged:
1⋅v=v
This mirrors the role of 1 in regular multiplication.
Example: Scaling Forces in Physics
Consider a physics scenario where a force vector represents the push or pull applied to an object. Let's denote this force vector as F:
F=[3−2]
Here, the vector F has two components (let's say, in Newtons), with a magnitude of 3 in the x-direction and -2 in the y-direction.
Now, let's introduce a scalar k that represents a scaling factor. If k is equal to 2, we want to examine the result of scaling the force vector by a factor of 2:
F′=k⋅F
Substitute the values:
F′=2⋅[3−2]=[6−4]
In this example, the vector F' represents the scaled force. The magnitude of the force has been doubled in both the x and y directions. This scaling factor, in a physics context, might indicate applying the force with twice the intensity.
Graphically, if the original force vector F was represented as an arrow from the origin to the point (3, -2) in a coordinate system, the scaled vector F' would extend to the point (6, -4), indicating the change in magnitude while maintaining the same direction.
3. Economics: Profit Margins
In economics, vectors can represent quantities like profits and costs. Scalar multiplication might be used to analyse the impact of scaling these quantities, such as determining the effect of a percentage change in costs on overall profits.
CBSE Class 11th Downloadable Resources:
| 1. CBSE Class 11th Topic Wise Summary | View Page / Download |
| 2. CBSE Class 11th NCERT Books | View Page / Download |
| 3. CBSE Class 11th NCERT Solutions | View Page / Download |
| 4. CBSE Class 11th Exemplar | View Page / Download |
| 5. CBSE Class 11th Previous Year Papers | View Page / Download |
| 6. CBSE Class 11th Sample Papers | View Page / Download |
| 7. CBSE Class 11th Question Bank | View Page / Download |
| 8. CBSE Class 11th Topic Wise Revision Notes | View Page / Download |
| 9. CBSE Class 11th Last Minutes Preparation Resources | View Page / Download |
| 10. CBSE Class 11th Best Reference Books | View Page / Download |
| 11. CBSE Class 11th Formula Booklet | View Page / Download |
Being in CBSE class 11th and considering the board examinations you must be needing resources to excel in your examinations. At TestprepKart we take great pride in providing CBSE class 11th all study resources in downloadable form for you to keep you going.
Below is the list of all CBSE class 11th Downloads available on TestprepKart for both Indian and NRI students preparing for CBSE class 11th in UAE, Oman, Qatar, Kuwait & Bahrain.
SAMPLE PRACTICE QUESTIONS OF SIGNIFICANT FIGURES :
Q1. What is the Multiplication of Vectors by Real Numbers?
Answer: Multiplication of vectors by real numbers is an operation that scales the magnitude and direction of a vector by a numerical factor. Each component of the vector is multiplied by the scalar.
Q2. How is Scalar Multiplication Represented Mathematically?
Answer: If v is a vector and c is a real number, then the scalar multiplication is represented as c⋅v. Each component of v is multiplied by c.
Q3. What Happens to the Magnitude of a Vector During Scalar Multiplication?
Answer: The magnitude (or length) of the vector is scaled by the absolute value of the scalar. If ∣∣∣v∣ is the magnitude of the vector v and c is a scalar, then ∣c⋅v∣=∣c∣⋅∣v∣.
Q4. How Does Scalar Multiplication Affect the Direction of a Vector?
Answer: The direction of the vector remains the same if the scalar is positive. If the scalar is negative, the direction is reversed, pointing in the opposite direction.
Q5. Can Scalar Multiplication Change the Null Vector (Zero Vector)?
Answer: No, scalar multiplication cannot change the null vector. Multiplying the null vector by any scalar still results in the null vector.

| Class 11th CBSE Physics Chapters |
| Chapter1: UNITS AND MEASUREMENTS |
| Chapter2: MOTION IN A STRAIGHT LINE |
| Chapter3: MOTION IN A PLANE |
| > Introduction |
| > Scalars and vectors |
| > Addition and subtraction of vectors – graphical method |
| > Resolution of vectors |
| > Vector addition – analytical method |
| > Motion in a plane |
| > Motion in a plane with constant acceleration |
| > Uniform circular motion |
| Chapter4: LAWS OF MOTION |
| Chapter5: WORK, ENERGY AND POWER |
| Chapter6: SYSTEM OF PARTICLES AND ROTATIONAL MOTION |
| Chapter7: GRAVITATION |
| Chapter8: MECHANICAL PROPERTIES OF SOLIDS |
| Chapter 9: MECHANICAL PROPERTIES OF FLUIDS |
| Chapter10: THERMAL PROPERTIES OF MATTER |
| Chapter12: KINETIC THEORY |
| Chapter13: OSCILLATIONS |
| Chapter14: WAVES |
| Class 11th CBSE Chemistry Chapters |
| Chapter1: SOME BASIC CONCEPTS OF CHEMISTRY |
| Chapter2: STRUCTURE OF ATOMS |
| Chapter3: CLASSIFICATION OF ELEMENTS AND PERIODICITY IN PROPERTIES |
| Chapter4: CHEMICAL BONDING AND MOLECULAR STRUCTURE |
| Chapter5: THERMODYNAMICS |
| Chapter6: EQUILIBRIUM |
| Chapter7: REDOX REACTIONS |
| Chapter8: ORGANIC CHEMISTRY – SOME BASIC PRINCIPLE AND TECHNIQUES |
| Chapter9: Hydrocarbons HYDROCARBONS |
| Class 11th CBSE Mathematics chapter |
| Chapter1: SETS |
| Chapter2: RELATIONS AND FUNCTIONS |
| Chapter3: TRIGONOMETRIC FUNCTIONS |
| Chapter4: COMPLEX NUMBER AND QUADRATIC EQUATIONS |
| Chapter5: LINEAR INEQUALITIES |
| Chapter6: PERMUTATIONS AND COMBINATIONS |
| Chapter7: BINOMIAL THEOREM |
| Chapter8: SEQUENCES AND SERIES |
| Chapter9: STRAIGHT LINES |
| Chapter10: CONIC SECTIONS |
| Chapter11: INTRODUCTION TO THREE-DIMENSIONAL GEOMETRY |
| Chapter12: LIMITS AND DERIVATIVES |
| Chapter13: STATISTICS |
| Chapter14: PROBABILITY |
| Class 8 Link soon |
| Class 9 Link soon |
| Class 10 Link soon |
| Class 12 Link soon |
Leave a Reply